Introduction Area Calculator: Comprehensive Guide with Examples

Calculating the area of different shapes and objects is a fundamental aspect of geometry and various practical applications. Whether you're working on a construction project, solving math problems, or simply curious about measurements, an area calculator can significantly simplify the process. This article provides an in-depth guide on area calculation, covering various shapes, the formulas used, and practical examples to illustrate the concepts.

Understanding Area Calculation

Area is a measure of the amount of space within the boundaries of a two-dimensional shape or surface. It is typically expressed in square units, such as square meters (m²), square feet (ft²), or square centimeters (cm²). Calculating the area involves determining how much space is enclosed by the shape’s edges. Different shapes require different formulas to calculate their areas, reflecting their unique properties.

Common Shapes and Their Area Formulas

Here’s a detailed overview of the formulas used to calculate the area of some common shapes:

1. Rectangle

The area of a rectangle is calculated by multiplying its length by its width. The formula is:

Area = length × width

For example, if a rectangle has a length of 8 meters and a width of 5 meters, the area is:

Area = 8 × 5
Area = 40 square meters

2. Square

A square is a special type of rectangle where all sides are of equal length. The area of a square is found by squaring the length of one of its sides. The formula is:

Area = side²

For instance, if a square has a side length of 4 meters, the area is:

Area = 4²
Area = 16 square meters

3. Triangle

The area of a triangle can be calculated using the formula:

Area = ½ × base × height

Here, the base is the length of the bottom edge, and the height is the perpendicular distance from the base to the top vertex. For example, if a triangle has a base of 6 meters and a height of 3 meters, the area is:

Area = ½ × 6 × 3
Area = 9 square meters

4. Circle

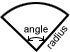
The area of a circle is determined using its radius. The formula is:

Area = π × radius²

π (pi) is approximately 3.14159. For example, if the radius of a circle is 5 meters, the area is:

Area = π × 5²
Area ≈ 3.14159 × 25
Area ≈ 78.54 square meters

5. Parallelogram

The area of a parallelogram is calculated similarly to a rectangle but involves using the length of the base and the height perpendicular to that base. The formula is:

Area = base × height

For instance, if a parallelogram has a base of 7 meters and a height of 4 meters, the area is:

Area = 7 × 4
Area = 28 square meters

6. Trapezoid

A trapezoid (or trapezium) has two parallel sides and two non-parallel sides. The area can be calculated using the formula:

Area = ½ × (base1 + base2) × height

Here, base1 and base2 are the lengths of the two parallel sides. For example, if a trapezoid has bases of 8 meters and 5 meters, and a height of 3 meters, the area is:

Area = ½ × (8 + 5) × 3
Area = ½ × 13 × 3
Area = 19.5 square meters

7. Ellipse

The area of an ellipse is found using the formula:

Area = π × semi-major axis × semi-minor axis

Here, the semi-major axis is half of the ellipse’s longest diameter, and the semi-minor axis is half of the shortest diameter. For example, if an ellipse has a semi-major axis of 6 meters and a semi-minor axis of 4 meters, the area is:

Area = π × 6 × 4
Area ≈ 3.14159 × 24
Area ≈ 75.4 square meters

Applications of Area Calculation

Calculating area is crucial in many real-life situations, including:

Construction: Determining the amount of materials needed for flooring, painting walls, or building structures.
Gardening: Calculating the space required for planting, designing garden layouts, or estimating the amount of soil needed.
Landscaping: Measuring areas for patios, driveways, and other outdoor features.
Real Estate: Assessing the size of properties and determining their value based on square footage.
Interior Design: Planning the layout of furniture, calculating space for decor, and optimizing room usage.
Science and Engineering: Calculating surface areas for experiments, designing equipment, or analyzing spatial data.

Examples and Practice

Let’s explore a few more examples to solidify the understanding of area calculation:

Example 1: Calculating the Area of a Room

Suppose you want to calculate the area of a rectangular room that measures 12 feet in length and 10 feet in width. Using the formula for the area of a rectangle:

Area = length × width
Area = 12 × 10
Area = 120 square feet

This means the room covers an area of 120 square feet, which helps in determining the amount of flooring or paint needed.

Example 2: Finding the Area of a Garden Bed

Consider a square garden bed with each side measuring 8 meters. To find the area, use the formula for the area of a square:

Area = side²
Area = 8²
Area = 64 square meters

The garden bed has an area of 64 square meters, useful for calculating the amount of soil or mulch required.

Example 3: Area of a Circular Pool

Imagine you have a circular swimming pool with a radius of 10 meters. To calculate its area:

Area = π × radius²
Area ≈ 3.14159 × 10²
Area ≈ 3.14159 × 100
Area ≈ 314.16 square meters

The pool has an area of approximately 314.16 square meters, which is essential for determining the volume of water needed.

Conclusion

Area calculators are invaluable tools that simplify the process of measuring and calculating the space within various shapes and objects. By understanding and applying the formulas for different geometric shapes, you can make accurate measurements for construction, gardening, real estate, and numerous other applications.

With practical examples and clear explanations, this guide aims to enhance your knowledge of area calculation, making it easier to apply these concepts in everyday scenarios. Whether you're a student, professional, or DIY enthusiast, mastering area calculations will empower you to make informed decisions and achieve precise results.